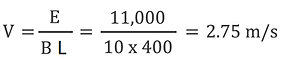Sim's Electrical Companion
Fleming's Left Hand Rule - The Motor Effect
Fleming discovered the relationship between Magnetic field strength, length of conductor, Force and current. The effect of this is when motors are being constructed they can be mathematically modelled first. The required force is the starting point for a designer and using this equation (simplified) a motor can be designed to achieve the task. This is albeit highly simplified but is still a fundamental starting block.
Left hand motor rule:
F = Force in Newtons (N)
B = Magnetic field strength in Tesla’s (T)
L = Length of conductor in meters (m)
I = Current in Amperes (A)
The mnemonic that I was taught in college is not repeatable in this forum but it did stick in my head. Instead I will offer a more PC version:

Force Brings Life Into - Motors
What this equation brings to designers is the tool to make choices based on the requirements of the motor. It would be crazy to have a V8 toothbrush and like wise a toothbrush motor may not be quite what you want to take a lift to the top of the Shard.
Motors come in all shapes and sizes and this is to suit the purpose that they are required to serve and with good design will provide this reliably for a reasonable amount of time. One area where motor design becomes tricky is when you require a large amount of force but the constraint is space.
Using this equation you could potentially create a very high torque motor in a small space if you reduce the conductor size you can increase the length of the conductor. Your design issue would then be cooling the undersized conductor. Another method would be to increase the power of the magnetic field.

The illustration (Right) shows the motor effect. It is dependant upon the direction of the magnetic field and current flow. These are depicted by a left hand's thumb, fore and index fingers being perpendicular to each other.
thuMb = Motion
Fore finger = Magnetic Field
SeCond finger = Current
Worked Example:
If a motor is required to attain a force of 300 Newtons calculate what current would be required if the winding is 30m and the magnetic
field density is 5 Tesla.
F = B L I
Rearrange the equation for current:
I = F / (BL) I = 300 / (5 x 30)
I = 300 / 150
I = 2 Amps
Fleming's Right Hand Rule Generator Rule
As well as developing a rule for the design of motors a similar set of rules apply to generators. Most simple motors will act like a generator if they are physically rotated. The voltage emitted is dependant upon the speed the motor is turned. For this reason it is sensible to not turn motors that are connected in case the current produced damages components that are connected to it. For this reason it is not a sensible idea to hoover a PC's case fans!
Right hand generator rule:
Fleming determined that like the motor rule the motion, field direction and induced current were perpendicular to each other. Remember Generator (riGht)
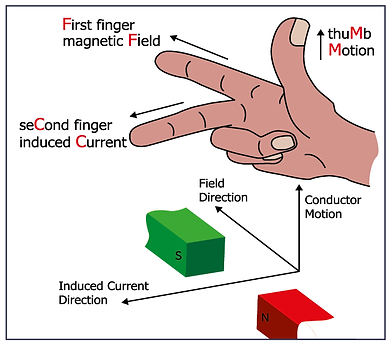
The ThuMb represents Motion
The Fore / First finger represents magnetic Field N-S
The seCond finger represents induced Current
The amount of energy produced can be determined by the following formula:
E = emf (electro motive force) Volts (V)
B = Magnetic field strength in Tesla’s (T)
L = Length of conductor in meters (m)
V = Velocity (m/s)
Once again the mnemonic I was taught for this was wrong on so many levels. A common mistake is to think of V as Voltage so I will offer this:This demonstrates that generated voltage is relative to the length of the conductor, strength of the magnetic field and the speed that the conductor cuts through the magnetic field.
Every Body Loves Velocity
In some circumstances size may be a limiting factor. To increase the generated emf the choices are to increase the magnetic field or the velocity of the conductor cutting across the magnetic field. (At the time of writing a boron neodymian magnet had a been created with a field strength of 34000kg!)
Opposite to that you may want a long service life so reducing the speed and associated wear on mechanical parts can be achieved by increasing the length of the conductor coil or strength of the magnetic field. A micro wind turbine is a good example of this application.
Worked Example 1
How much voltage would be generated if a 25m conductor cuts across a 4 Tesla magnetic field at a rate of 2 metres per second?
Worked Example 2
What speed would be required to produce 11kV if the winding is restricted to 400m with a magnetic field density of 10 Tesla?
Rearrange the formula for Velocity: